In the introduction to the Xin zhi lingtai yixiang zhi, Verbiest emphasized the importance and justification for the making of six new astronomical instruments, stating that the elaboration of a correct calendar required correct observations, these only being possible with the assistance of adequately calibrated instruments.
To justify his demand, he referred to the observations and calculations made in the presence of Tu Hai and Li Wei, on the 3rd of February 1669. These official observations for fixing the li chun were printed in both his Ce yan chi lue and Astronomia Europœa. They were mentioned for the first time in Verbiest's Ce yan chi lue, edited in 1668, and later in the Astronomia Europaea edition of 1687. 1
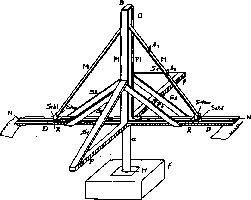
Both in the Ce yan chi lue and the Astronomia Europœa Verbiest only alludes to the use of the quadrant and sextant for the register of the above mentioned observations; but already in the Yixiang zhi he presented calculations registered by six instruments: [1.] the Ecliptic Armillary instrument, [2.] the Equatorial instrument, [3.] the Azimuthal instrument, [4.] the Quadrant, [5.] the large Sextant and [6.] the Celestial Globe.
As the new astronomical instruments were erected between 1669 and 1673 Verbiest did not use the new instruments for the calculations, having probably solved the calculations by trigonometry.
 Ecliptic armillary instrument.
In: VERBIEST, Ferdinand, Yixiang tu, ill.1.
Ecliptic armillary instrument.
In: VERBIEST, Ferdinand, Yixiang tu, ill.1.
A petition of the 10th of September 1669 (KX 8:8:16) seems to refer to the probable beginning of the construction of the instruments. In this petition Verbiest asked the Kangxi Emperor permission to have the instruments built. In the introduction to his later exposition on the newly-built instruments there is a reference to the 'Autumn' of the same year to begin the proposed construction. In the compendium of the Liber Organicus, he mentioned that he had the six instruments built over a four year period. 2
In a letter of the 20th of August 1670, addressed to Jacques Le Faure, he gave indications of how the instruments were then being built: "I am sending your Reverend designs or sketches of astronomical instruments, which the tribunal Kongbu, on the basis of my prescribed prototype, has taken the responsibility to construct of the best brass, with the purpose of placing them in the "mathematical tower" [ie: the Observatory] as soon as the old ones have been removed. I call them sketches because these designs do not completely correspond with those instruments which have already been half-worked out and which, with God's help, must be completed within this year."3
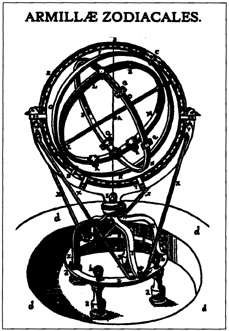 Armillœ Zodiacales (Ecliptic armillary instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.52 -detail.
Armillœ Zodiacales (Ecliptic armillary instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.52 -detail.
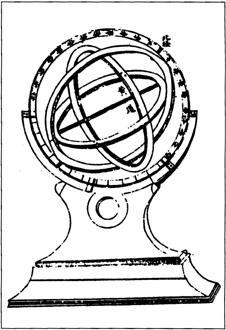 Ecliptic armillary instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p. 737-detail.
Ecliptic armillary instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p. 737-detail.
It is clear from this letter that Verbiest hoped to have finished the instruments before the end of 1670, but from a quotation from a letter of De Rougemont4 it appears that they were not finished until 1673. In this same letter he also indicated that the construction of the instruments was by the Kongbu (Ministry of Works). 5 This fits in with the data of the edicts which mentioned that the erection of the astronomical in struments was started in the eighth year (1669/ 1670) (KX 8:8:1; 26th of August 1669), but was not finished until the thirteenth year (1674).
More exact data regarding the construction of the new instruments' evolution is to be found in a petition of the Kongbu. This petition mentioned that in the tenth month of the eighth year (October-November 1669), Verbiest was to have at his disposal the materials needed for casting the six bronze instruments as well as for the making of the cast iron fence. The Ministry of Finance was asked for a financial contribution of 7,484.73 taels for jinye (gold-leaf), tong (bronze), tie (iron), huang la (yellow wax), songxiang (resin) and the wages of the craftsmen. In the third month of the tenth year (March-April 1671) he asked a sum of 379.44 taels for supplementary expenses of materials and craftsmen. In the fourth month of the eleventh year (April-May 1672) he asked 3,454.90 taels extra for the cast iron fence, more exactly forthe coal needed to cast it. In the first month of the twelfth year (January-February 1673) Verbiest asked 500.30 taels for the gilding of the stars (on the Celestial globe) and 656.98 taels for supplementary material (coal), for the cast iron fence and the wages of the craftsmen. At the end of the same year of 1673, the work was finished and the instruments were to be placed each one in their own place in the Observatory. We can clearly accept that the instruments were completed by the end of 1673.
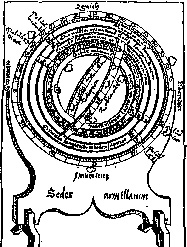 Ecliptic armillary instrument.
In: REGIOMONTANIS, Ioannis M., Scripta Cla-/rissimi Mathemati-/ci [...], Norimbergæ, apud Ioannem Montanum & Vlricum Neuber, 1544 - detail.
Ecliptic armillary instrument.
In: REGIOMONTANIS, Ioannis M., Scripta Cla-/rissimi Mathemati-/ci [...], Norimbergæ, apud Ioannem Montanum & Vlricum Neuber, 1544 - detail.
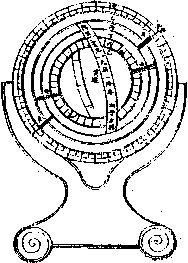 Ecliptic armillary instrument.
In: RHO, J., Ce liang quan yi, 1631, SKQS 789, p. 727 - detail.
Ecliptic armillary instrument.
In: RHO, J., Ce liang quan yi, 1631, SKQS 789, p. 727 - detail.
Verbiest emphasized the use of different instruments to have a more reliable verification. He also justified the construction of different new instruments by comparing them with the yuan instruments of Guo Shoujing:
"Suppose we restricted ourselves to one instrument to investigate everything in the sky, like, for instance, the jian yi (compendium instrument) of the old method. On what grounds could we trust its accuracy? Among all instruments the celestial globe is the instrument which shows most resemblance to the celestial sphere and which has most applications. There is none equalling the celestial globe. For the globe is a complete model of the celestial sphere and it combines the applications of all instruments in itself"6
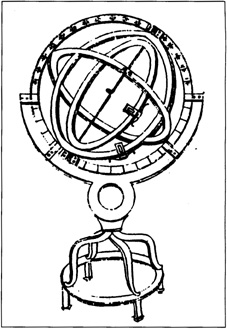
Tycho Brahe's instruments, as described and represented in the Astronomiœ instauratœ mechanica (1598), were the prototypes for the construction and description of Verbiest's newlybuilt bronze instruments.
[1] For the Ecliptic Armillary instrument, Verbiest used Tycho Brahe's Armillœ Zodiacales, 7 a simplified model constructed with four rings instead of the classical Hellenistic model constructed with five or six rings. 8
In spite of the fact that in his description Brahe himself rejected this instrument when the greatest precision was required:
 Armillœ Aliœ Equatoriœ (Equatorial instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.56 - detail.
Armillœ Aliœ Equatoriœ (Equatorial instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.56 - detail.
 Armillœ Aliœ Eqvatoriœ (Equatorial instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in. "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.60 - detail.
Armillœ Aliœ Eqvatoriœ (Equatorial instrument).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in. "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p.60 - detail.
"Atque hinc factum, ut rarius talibus armillis praesertim cum summa praecisio requiritur, uteremur. Quin potius alias aequatorias armillas excogitavimus, huic vitio non obnoxias. Quod etiam nullo modo in majoribus zodiacalis emendari potest, ob eo gravius armillarum pondus",9 and J. Hevelius in his Machinae coelestis (1673) rejected all the armillary instruments as useless for serious matters: "Ad res serias armillae haud sunt adhibendae".10 Verbiest still reconstructed an ecliptic armillary instrument, but he bore in mind the difficulties and the weaknesses Brahe mentioned with regard to his own instruments, and made technical improvements. He added a support ring to improve the stability of the instrument. That Verbiest paid attention to stability was confirmed in a letter to Jacques Le Faure, dated the 20th of August 1670:
"Dans la confection du support ou pied, j'ai cherché avant tout à obtenir une ferme et bonne adhésion au sol, de manière à supprimer toute trépidation des instruments"11 and in Chapter Two he inserted a Treatise on Stability.
But it was not the first time that this instrument of Tycho Brahe was mentioned and illustrated in the Chinese Jesuitic texts. The Ce liang quan yi described the same model under the title: Xin fa Huangdao jingwei yi (New Method for the Ecliptic Instrument). 12This Chapter concisely dealt with the description of the different parts of the instrument.
[2] The second instrument Verbiest described is the Equatorial Instrument. If we compare Verbiest's construction with the different types of Tycho, we can say that Verbiest did not choose the most simply constructed equatorial instrument of Tycho, which was the Armillae aequatoriae maximae, but the type with three rings, where the Equatorial ring was fixed.
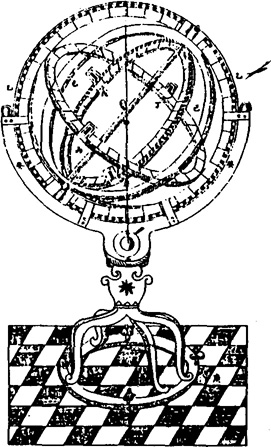 Equatorial instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p.745 - detail.
Equatorial instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p.745 - detail.
 Equatorial instrument.
In: RHO, Johannes; SCHALL, Adam, Heng xing lizhi, SKQS 788, p.975 - detail
Equatorial instrument.
In: RHO, Johannes; SCHALL, Adam, Heng xing lizhi, SKQS 788, p.975 - detail
If we compare Verbiest's instrument with that of Guo Shoujing, then it was a simpler construction.
Tycho Brahe's Equatorial instruments were also mentioned before in the Ce hengxing chidao jing wei du zhi qi. This Chapter briefly describes the different parts of the instrument and its method of use.
The illustration is the Armillœ aliœ aequatoriœ. 14
Under the heading: Xin fa chidao jing wei yi15 the Ce liang quan yi also includes a description of two different types of Tycho Brahe's Equatorial instruments, namely: the Chidao jing wei jian yi (Armillae œquatoriœ maximå16 or, Simple Equatorial Instrument) and Chidao jing wei quan yi (Armillœ alice œquatoriae 17, or, Complete Equatorial Instrument ). The Chinese description of the latter one corresponds with Verbiest's description but does not describe the corresponding instrument of Tycho Brahe. The Chidao jing wei quan yi included in the Ce liang quan yi corresponds to the description and illustration of the Ce hengxing chidaojing wei du zhi qi, namely the Armillœ aliaœ œquatoriœ or an instrument with four armillœ, the equator of which is movable.
The Equatorial instrument of Verbiest corresponds with Tycho Brahe's instrument called Armillœ œquatoriœ, 18 or an instrument with three rings, the quator of which is fixed.
In the description of his instruments, Verbiest gave us detailed explanation about the joining together of the different parts.
Different from the construction of Tycho Brahe is that Verbiest added a supporting half ring.
The illustration differs from Tycho's, as Verbiest gave us a special position of the instrument, and he observed: "When turning this [declination ring] in the East-West direction, it skims [inside] the Equator ring."19 This special position of the declination ring was also mentioned by Tycho Brahe: "[...] quae etiam aliquando plano meridiani uniri potest".20
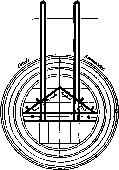
The Azimuthal instrument is the only described and constructed instrument of which we have no prototype in Tycho Brahe's Astronomiœ instauratae mechanica, although Brahe describes different Azimuthal quadrants.
In his introduction Verbiest mentions three different types of construction, which are based on the chosen coordinate system. Important is that Verbiest refers to the third type, based on the Azimuthal coordinate system, as "Altazimuthal instrument", which can further be divided into the Azimuthal instrument and the Altitude instrument which is in fact a Auadrant, but which is easier to use. 21
This is the only reference to explain why he did not construct an Altazimuthal instrument, but both an Azimuthal instrument and a Quadrant, although his predecessors, Rho and Schall, described and illustrated the Altazimuthal instrument as a new instrument. The method of using this latter instrument was recorded in the Ce liang quan yi under the heading: Xin fa diping jiping wei yi (New Method for the Azimuthal and Altitudinal Instrument). 22
 Equatorial instrument.
In: VERBIEST, Ferdinand, Yi xiang tu, ill. 2.
Equatorial instrument.
In: VERBIEST, Ferdinand, Yi xiang tu, ill. 2.
In 1713, K. Stumpf constructed a new Altazimuthal instrument, in succession to Verbiest. In his 1744 report concerning the examination of the instruments, elaborated by order of the Kangxi Emperor, Ignatius Kögler mentioned that Stumpf's was ordered by the Emperor to construct the new instrument. In the same report he expressed an unfavourable opinion on Verbiest's use of mutually combined in struments. 23 The separate use of an Azimuthal instrument can be found in the devised instruments of Guo Shoujing. His jian yi (simplified instrument) can be considered as an opened-out instrument with separate components such as the so-called li yun xuan (revolving vertical circle), composed of a fixed Azimuthal ring and a revolving circle.
On the other hand the Chinese (or Sino-Korean) concept of Verbiest's instrument, with the use of oblique threads, was first mentioned and explained in The Hall of Heavenly Records. 24
Verbiest's trigonometrical approach, and his reference in the Ji he yuan ben, to Euclidian geometry, when he discusses the accuracy of the instrument, 25 equally resembles the Maragha instrument, called "the instrument for the Sinus and the Azimuth."26
Both Maragha and Verbiest's instruments have a vertically-mounted bar in the centre of the horizontal ring - in the first example, with a rectangular opening containing two bars, and in the second example, with two oblique threads, but with the same use. For measuring and alignment, both refer to a method of ninety-degrees triangles.
What is beyond doubt is that, whenever possible, Verbiest attempts to achieve some kind of conformity with the Chinese instruments, which enables him to defend more efficiently his own instruments against the existing Chinese- Yuan instruments. Maybe this was the reason why he chooses a separate Azimuthal instrument.
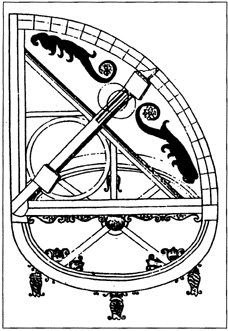
[4] The Quadrant being unknown to the Chinese, its construction was an important revelation. The Chinese generally used the gnomon to measure altitudes. It is therefore not surprising that this instrument is dated and bears Verbiest's signature. With the calendar reform of 1629, the Jesuits Johannes Rho and Adam Schall introduced the Quadrant as one of the newly acquired instruments. 27 While Rho and Schall's benchmodel Quadrant derived from Tycho Brahe's Quadrans minor orichalcicus inauratus, 28 Verbiest's model derives from Brahe's Quadrans volubilis azimuthalis. 29
This instrument is again an example of how Verbiest tries to achieve a kind of conformity with similar Chinese-Yuan constructions. His description states that: "The inner space of the Quadrant is filled with a flatly elaborated dragon, fastened to all sides"30 Verbiest's reason for adding a "Chinese dragon" and a geometrical framework" to Brahe's original instrument is not explained by him but, apparently, has the same function, as it can be inferred from Brahe's writings:
"Contignationes, quae intra quadrantis aream cernuntur, sunt propterea in eum modum multifariam dispositae, ut illum firmiter tam in suo plano quam reliqua conformatione debita retineant atque conservent."31
 Azimuthal instrument.
In: VERBIEST, Ferdinand, Yi xiang tu. ill. 3.
Azimuthal instrument.
In: VERBIEST, Ferdinand, Yi xiang tu. ill. 3.
It is also important that Verbiest refers to Euclidian geometry in the Ji he yuan ben to explain the method of measuring an angle within a circumference, illustrating it with a plate (no 13). In Chapter Two this method is further discussed at length. The mounting of the instrument, especially the mounting of its vertical axis, as described in the Yi xiang zhi, deviates from the model erected at the Beijing Observatory, but corresponds with its description in the Astronomiœ Europœa. The discrepancies between the book illustrations and the Observatory's construction were mentioned by Verbiest in a letter to Jacques le Faure, dated the 20th of August 1670. 32
[5] Like the Quadrant, the Sextant was unknown to the Chinese. It was introduced for the first time with the calendar reform of 1629, being one of the newly acquired instruments by Rho and Schall. 33
In Chapter One of the Ce hengxing xiang ju zhi ji, 34 an illustration of Brahe's Sextans astronomicus trigonicus pro distantiis rimandis35 is reproduced its corresponding explanation (ie: the instrument's method of use) being a transcription of Brahe's original text. As he clearly stated, Brahe considered himself as the inventor of the Sextant:
"Sextantis astronomici instrumentum usibus apprime accomodatum ego primus ante annos plus minus 20. excogitavi, adeoque hoc nomen ipsi indidi, quod sextam circuli partem in circumferentia complectatur".36
In this case the Jesuits could boast of having introduced a new instrument.
In his Machinœ coelestis, Hevelius emphatically recommended the use of bronze casted sextants and declared himself surprised since such a rich man as Brahe had not followed this practise; he then gave the reason for Brahe's non compliance: "Genuina autem causa, quare id non fecerit Tycho [Brahe], haec est quod omnino in ea fuerit opinione, sextantes & octantes ex metallo tanto magnitudine fabrefactos, nullo modo à duobus observatoribus, prout merito debet, fore tractabiles, multo minus ad nutum quaquaversum flexibiles; fit ut hac ratione instrumentorum moles, & pondus exoptatissimo effectui omnino resisteret. Optimè tamen perspexit, organa metallica longè fore excellentiora, & securiora pro observationibus coelestibus, dummodo leviusculo labore tractari, ac expeditè regi illa concederetur."37
 Azimuthal instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p.740 - detail.
Azimuthal instrument.
In: RHO, Johannes, Ce liang quan yi, 1631, SKQS 789, p.740 - detail.
The problems Brahe found in the construction of the Sextant (also mentioned by Hevelius), namely, the instrument's stability and its handling difficulty by only two persons, were reconsidered by Verbiest. For stability precautions he strengthened the instrument, introducing a triangularly shaped reinforcement, both to the right and left sides, above and below: "moreover the instrument is, to the right and the left, strengthened by interconnected little clouds, which account for its ultimate solidity".38 In this way the instrument was strengthened while kept as light as possible, whilst at the same time enabling precise celestial readings without incurring into deviations. Concerning the handling of the instrument, Verbiest devised a mechanism which was able to tilt it into three directions and adjust settings requiring a fraction (about one fifth) of the customary leaverage. Verbiest recurred to three small columns devised in order to align the instrument's orientation, one at the centre, the other two symmetrically placed at both its right and left sides. The diameters of the columns placed to the right and left were ten degrees off the bar's axis line. This system allowed observations to be made simultaneously by two people, one aligned with the side pinnule at the end of the alidade via the little round cylinder and the other aligned with an adjustable pinnule via the central cylinder. 39
Comparing Verbiest's Sextant with Brahe' s instruments, the construction of the former shows most resemblance with the latter's Arcus bipartitus minoribus siderum distantiis inserviens. 40 To be more precise, Verbiest's Sextant system is a combination of Brahe's Arcus bipartitus and his Sextans astronomicus trigonicus pro distantiis rimandis. 41
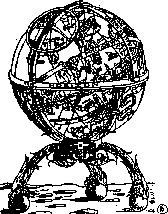
Western Globes were introduced in China by Matteo Ricci. The Opera storiche42 mentions that, in 1586, Ricci had three Globes constructed. According to Henri Bérnard-Maître these Globes must have been constructed on the model of those in the Collegio Romano. 43 Some of the Collegio Romano's Globes date from ca1570, and belonged to Hieronymo de Boncompagni. 44 In the memorial of the 13th of September 1629, Xu Guangqi proposed to manufacture astronomical instruments including a Celestial Globe with longitudinal and latitudinal lines. 45
In the Xi fa li yin (Ce qi)46 and the Xin fa biao yi, 47 Rho and Schall listed the new instruments that were acquired by the Astronomical Bureau. This list included a tian qiu (Globe). 48
 Azimuthal instrument.
In: SEDILLOT, L. A., Mémoire sur les instruments astronomiques des Arabes, in: "Memoires présentés par divers savants à l'Académie Royale des Inscriptions et Belles-lettres de l'Institut de France", Paris, 1844.
Azimuthal instrument.
In: SEDILLOT, L. A., Mémoire sur les instruments astronomiques des Arabes, in: "Memoires présentés par divers savants à l'Académie Royale des Inscriptions et Belles-lettres de l'Institut de France", Paris, 1844.
 Azimuthal Instrument.
In: SEDILLOT, L. A., Mémoire sur les instruments astronomiques des Arabes, in: "Memoires présentés par divers savants à l'Académie Royale des Inscriptions et Belles-lettres de l'Institut de France", Paris, 1844.
Azimuthal Instrument.
In: SEDILLOT, L. A., Mémoire sur les instruments astronomiques des Arabes, in: "Memoires présentés par divers savants à l'Académie Royale des Inscriptions et Belles-lettres de l'Institut de France", Paris, 1844.
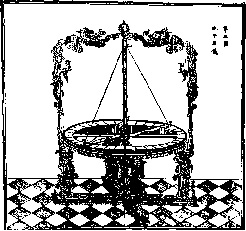
In Chapter Five of the Xin fa suan shu, it is recorded that, after January 1635, amongst some important instruments, there was a huntian yi. 49
In a letter from Fr. Furtado to the Visitor, 50 from Beijing, dated the 25th of June 1673, the author makes detailed references to this globe. Further information is given by D. Bartoli. 51 Bérnard supposed that the information was based on a letter of Rho where he painstakingly described other features of the Globe, such as the ecliptic indicating on the twelve signs, the twenty-eight characters pertaining to the zodiac, and twenty-eight other characters related to the lunar configurations. This celestial globe of Schall may have been the prototype of Verbiest's globe. Chapter Five of the Huntian yi shuo 52 contains a detailed description of Schall's globe. The different possibilities for the use of the globe are described by Schall in Chapters Two, Three and Four, and most of these possibilities are transcribed by Verbiest in Chapter Two of his work, but not as extensively explained as in the former' s text, the author not dealing with technical details. For instance, is not mentioned by Verbiest, but extensively explained. In the Huntian Yi Shuo Chapter Five, Schall deals with the method of placing the stars on the Globe's spherical surface, in Verbiest's analysis of the Globe analysis, its structure and its two external rings (the meridian and the horizontal) are described right from the start as already construed. Technical data explaining Verbiest's Globe construction system are only be found in his correspondence.
 Alivd Instrvmentvm Simile Priori, Pro Distantiis (Arch of altitude)
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p. 102 - detail.
Alivd Instrvmentvm Simile Priori, Pro Distantiis (Arch of altitude)
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia [...]", 15 vols., Hauniæ, Det Danske Sprog- og Litteratur-Selskab, vol.5, p. 102 - detail.
 Gao hu. (Arch of altitude) Globe by J. Schöner.
Gao hu. (Arch of altitude) Globe by J. Schöner.
In a letter dated the 20th of August 1670, to Faure, Verbiest remarked that he had first built a full-scale wood model of each instrument and that these models were afterwards sent to the Kongbu (Ministry of Works). 53 Whereas the descriptions of most of Verbiest's instruments closely follow their corresponding descriptions in Brahe's Astronomiœ instaurate mechanica, the description of his Celestial Globe has greater similarities with that of R. Hues, in his Tractatus de globis et eorum usu. Hues Treatise was first published in London, in 1593, to be followed by many reprints and editions. In the seventeenth century it was a useful standard reference work for the making and practicalities of Globes.
[6] Writing on the Celestial Globe, Verbiest explained the use of two kinds of segments of a bian quan si fen zhi yi (circle), namely, the gao hu (arch of altitude) - mounted in the zenith - and the wei hu (arch of latitude) - mounted on the ecliptic or equatorial pole. The arch of altitude is also mentioned and discussed by Hues in his Tractatus. 54 Brahe also mentioned the use of this altitude segment to define the measurement of an altitude and of the azimuth. 55 The arch of latitude is, apparently, only referred to in passim, in Johannes Regiomontanis' Epytoma Joannis de Monte Regio in Almagestum Ptolomei. 56 The Beijing old Observatory bears no trace of these segments. At the end of the narrative on the Celestial Globe, Verbiest mentioned constellations and nebulae that can only be observed by telescope, concluding with a reference to the publication of two of his Celestial Charts.
After the description of the six-newly built instruments, Verbiest explained in detail their Observation and Calibration Methods. Under the heading Kui bao (Pinnule Observation) he described their observation method. This method has close associations with the Yi qi tu shuo, 57 the Chapter Ten of the Ce liang quan yi. Bearing in mind that Verbiest considered the pinnule as one of the 'observation methods' and in view of this text's content, the heading Kuifa (Observation Method), adopted by Rho and Schall, would have been a more appropriate one. Maybe Verbiest called it Pinnule Observation to emphasise a contextual correspondence with Brahe's previous exposition on the same subject, although, he does not describe the same pinnule system of Brahe.
Van de Vertical Cirkel: Of Quadrans Altitudinis
 Gao hu (Arch of altitude)
In: HUES, Robertus, Tractaet Ofie Handlinge van het gebruijck der Hemelscher ende Artscher Globe. [...] In 't Latijn eerst be∫chreven / door Robertum Hues, Mathematicum, nu in Neder-/duytsch overgeset/ ende met diversche nieuwe verclaringhe ende Figuren vermeerdert en verciert. Door I. Hondium, Amstelredam, Michiel Colijn, 1612, p.5.
Gao hu (Arch of altitude)
In: HUES, Robertus, Tractaet Ofie Handlinge van het gebruijck der Hemelscher ende Artscher Globe. [...] In 't Latijn eerst be∫chreven / door Robertum Hues, Mathematicum, nu in Neder-/duytsch overgeset/ ende met diversche nieuwe verclaringhe ende Figuren vermeerdert en verciert. Door I. Hondium, Amstelredam, Michiel Colijn, 1612, p.5.
 Quadrans Maximus Chalybevs Quadrato Inclvsvs, et Horizonti Azimuthali chalybeo, in∫istens (Brahe's method of calibration).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia[...]", 15 vols., Hauniæ, Dot Danske Sprog- og Litteratur-Selskab, vol.5 p.153.
Quadrans Maximus Chalybevs Quadrato Inclvsvs, et Horizonti Azimuthali chalybeo, in∫istens (Brahe's method of calibration).
In: BRAHE, Tycho, Astronomiœ instauratœ mechanica, in: "[...] Opera Omnia[...]", 15 vols., Hauniæ, Dot Danske Sprog- og Litteratur-Selskab, vol.5 p.153.
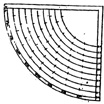 Nonius' Method of Callibration.
In: RHO, Johannes., Ce liang quan yi, 1631, SKQS 789, p. 737.
Nonius' Method of Callibration.
In: RHO, Johannes., Ce liang quan yi, 1631, SKQS 789, p. 737.
 Method of Calibration.
In: HENRION, Denis, L' Vsage du compas de proportion, Paris, Charles Hvlpeav, 1626.
Method of Calibration.
In: HENRION, Denis, L' Vsage du compas de proportion, Paris, Charles Hvlpeav, 1626.
Chapter One ends with short descriptions about the instruments' handling methods. These methods are generally taken from Schall and Rho. 58
[II] Chapter Two starts with instructions for the handling of the six instruments, these instructions being also adapted from Rho and Schall's previous writings. Verbiest succintly describes his instruments' multiple purposes, but for further detailed explanations refers to the work of Schall. 59
Chapter Two contains a series of Treatises about the practical application of his instruments.
Chapter Three especially emphasizes the advantages of his six instruments in comparison to those of Guo Shoujing, particularly elaborating on the Chinese yuan hun yi (Armillary instrument) and jian yi (Simplified instrument)
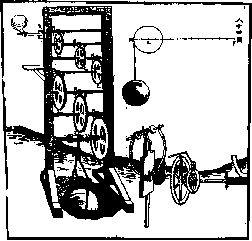 Power of transmission.
VERBIEST, Ferdinand, Yi xiang tu. ill. 84.
Power of transmission.
VERBIEST, Ferdinand, Yi xiang tu. ill. 84.
The presence of the old Chinese-Yuan instruments in the Beijing old Observatory and Verbiest's request to remove them is confirmed by the Imperial Edicts of the 26th and 28th of August 1669 (respectively: KX 8:8:1 and KX 8:8:3). 60 In order to convince the Emperor that the old instruments had become technically obsolete, Verbiest had to emphasise the importance of his new instruments for obtaining accurate celestial observations.
In the First Treatise he underlines the imprecise readings obtained from Guo Shoujing instruments' double ring system and stressed the complexity of their components as being a hindrance for gathering accurate observations and extrapolating correct calculations.
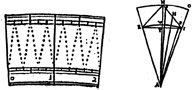
In the Second Treatise he discusses the importance and reason why, in order to have a more detailed calibration, more space is required and, therefore, the importance for implementing large-scale instruments. 61 He debates this, based in Euclides Elements, Book Three, Proposition 20: "In a circle, the angle at the centre is double of the angle at the circumference, when the angles have the same circumference as base". This method had been previously explained by Brahe in his description of the Semicirculus magnus azimuthalis. 62
Verbiest terminates with a detailed Treatise about his specific method of calibration employing transversals. His transversal method of calibration followed the Nonnius method; it would later be replaced by the Vernier method.
Verbiest's Calibration method, although merging the full lines of Bürgi to the basic principles of Brahe - and thus indicating a synthesis of the Bürgi and Brahe methods - seems to have been most probably inspired by Henrion's calibration method used on the 'mecometre' described and illustrated in this author's L' usage du mecometre, published in Paris, in 1630. Particularly interesting is that Verbiest's Yi xiang tu illustration no 5163 is copied from an illustration of Henrion's book. Verbiest concluded the Treatise with a comparison between his method of Calibration with the one used on the Chinese- Yuan instruments, once again reiterating the superiority of his own instruments.
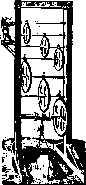 Power of transmission.
In: CAUS, Salomon de, Les Raisons Des Forces Mouvantes Avec diver∫es Machines Tant Utilles que plaisantes Aus quelles sont adioints plusieurs de∫eings de grotes et fontaines, [...], 3 parts, Francfort, Jan Norton, 1615, part. 1, bk. 1, theorem XVI, ill.8.
Power of transmission.
In: CAUS, Salomon de, Les Raisons Des Forces Mouvantes Avec diver∫es Machines Tant Utilles que plaisantes Aus quelles sont adioints plusieurs de∫eings de grotes et fontaines, [...], 3 parts, Francfort, Jan Norton, 1615, part. 1, bk. 1, theorem XVI, ill.8.
In his Third Treatise, on the bending moment and resistance to fracture, Verbiest follows Galileo Galilei's explanations of 'the first and second day' recorded by this scientist in his Discorsi e dimonstrazione matematiche. 64 After the shortgewu qiongli (philosophical introduction), intented to criticise coeval Neo-Confucianism doctrines, Verbiest proceeds to justify his dissertation concerning the essence of things and their fathoming principles. He explicitly extolls the Xi shi jia li le zhi fa (Method of the Western Scholar Galilei), following to explain Galilei's description of the "first day"; then he reveals some of the scientist's propositions of the "second day", namely: in succession, the third, first, fourth and eighth. Verbiest literally mentions Galilei's propositions, explaining his commentaries about them with examples. All examples except for that of proposition eight are correct. This attests for Verbiest's vast knowledge extolled to his systematic descriptive capacity.
The Fourth Treatise: Xin yi qing zhong bili fa (Method to Determine the Weight Ratios of the New Instruments), is particularly concerned with specific gravity. Its complete text, together with its comparative chart indicating the weight of bodies made of different materials, derived from the descriptions given on the same subject in M. Ghetaldi's Promotus Archimedes, published in Rome, in 1603, and Athanasius Kircher's Mundus Subterraneus, published in Amsterdam, in 1665.
Johann Schreck (Terrentius), in his Yuan xi qi qi tu shuo (Collected Diagrams and Explanations of Wonderful Machines from the Far West), published in 1627, was the first Jesuit who referred to specific gravity. In the Chapter Zhong jie (Explanation of Volume), Section Five (p.74), the author mentioned thewu zhi ben zhong (specific gravity). Sections Forty to Forty-five deal with some practical problems; Section Forty-five gives the same figure for the specific gravity for lead as the figure given by Verbiest in the Fourth Treatise
Important within this context is the Bi li gui jie (Explanation of the Compasses of Proportions), 65 an original text by Johannes Rho, published in 1630 in a revised version by Adam Schall. This work by Rho was based on Galilei's Le operazione del compasso geometrico e militare, published in Padua, in 1606. These compasses were six lines, the sixth of which was called the 'metal-line' and used to draw up the comparative chart.
The Bi li gui jie's comparative chart corresponds to that of Verbiest, their only difference being the ratio for quicksilver in relation to tin. The ratio given by Verbiest corresponded with that given by Ghetaldi and Kircher, the latter's figure most probably being the source for Verbiest's example. Verbiest reinforced his explanation with a practical example, giving the ratio for bronze in relation to other materials; also adding further important technical information for the manufacture of bronze instruments: "fan zhu tong yi. Xian yong la zuo ke yi zhi shi yang" ("in order to cast any bronze instrument one must previously make a wax model for it"). As in Ghetaldi's book, the double entries of the convertion chart were equally explained by Verbiest.

Mehod of Calibration.
In: VERBIEST, Ferdinand, Yi xiang tu, ill. 13.
The Treatise dealing with the importance of the centre of gravity for the construction of his improved instruments starts with a short account on the basics of geocentricism extracted from Sabbatino De Ursis Biao du shuo's66 Problem Four where the author returns to this subject in order to prove the sphericity of the earth. Exactly the same text and illustrations were also included in Verbiest's Bu de yi bian (1669) and Kunyu tu shuo (1672).
The importance of the centre of gravity for machinery is equally explained by Galilei in his Le Meccaniche (1593-1600): "Afin d'en tirer les causes et raisons de tout ce qui arrive aux Machines, dont il faut expliquer les effects, car chaque science a ses définitions & ses principes [...]Or puisque les Machines servent ordinairement pour transporter les choses pesantes, nous commençons par la définition de la pesanteur, que l'on peut aussi nommer gravité."67
To explain the gravitational pull towards the centre of the Earth Verbiest followed some passages of Terrentius' Yuan xi qi qi tu shuo text(p.73).
After this more theoretical dissertation on the centre of gravity, Verbiest continued with a more practical explanation punctuated by examples which correspond to that in Kircher's Mundus subterraneus. 68 In the Yi xiang tu Verbiest completed his exposé with illustrations reproduced from S. Stevin's Beginselen der Weeghconst.
The following section of Verbiest's text, mainly explained via illustrations, is the bronze casting of the instruments' different integral components. This section's framework and its underlying concept has affinities with Song Ying Xing's Tian gong kai wu (Exploitation of the Deeds of Nature), also written in the seventeenth century.
Precision implying an effective adjustment of each and all constituent parts of any precision equipment, Verbiest made use of efficient technical machinery such as the pulley, the gear-wheel and the screw, in the construction of his instruments.
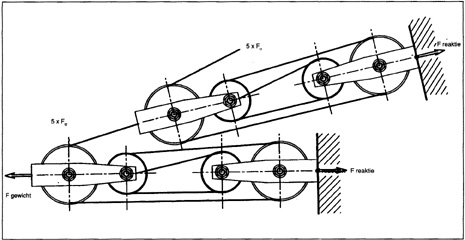
For the discussion on the pulley, Verbiest borrowed data and illustrations from Bettini's work69 and followed Paolo Casati's excursus on the 'trochlea multiplices'.70
The commentary on the gear-wheel -which he called lun xiang lian (wheels that engage one another) - was explained with examples complemented by illustrations previously published by De Caus. 71 It is not surprising that Verbiest dedicated particular care to this section of the text as he used gear-wheels to effect the powered-transmission rotation of his celestial globe and sextant.
Verbiest concluded Chapter Two with some notes about the screw, based on Galilei's more elaborate study on this subject, from Le Meccaniche, printed ca 1600.
It is a fact that correct observations depend from the "precise" assembly of the instruments' components.

Calibration method.
In: VERBIEST, Ferdinand, Yi xiang tu, ill.63.
Before Verbiest progressed in his text supplying information on the "precise" assembly of his instruments', he carefully introduced the reader to the accurate meaning of the literary terminology he was about to employ. According to him, "precise" instrumental observations, meant foremost, ascertaning the location of the magnetic North or determining the needle's deviation angle from the geographic North. The absolute necessity to define this distinction was crucial when Verbiest ascertained the deviation of the East-West wall of the Beijing Observatory.
Verbiest's elaboration on this topic and on its close connections to the Earth's magnetic forces generally followed the line of thought expressed by Riccioli in Book Nine of his Almagestum Novum, 72 althought, his denser explanations on the compass variations are more according to Kircher's exposition on this matter. 73 For the method of determining with precision the North-South axis, Verbiest refered to the Rizhan lizhi. 74
After these preliminary commentaries Verbiest then explained the precise assemby of his instruments while insisting for their construction on the use of the plumb line and on the fixed North-South reference axis. These practical instructions are Verbiest's personal suggestions.
[III]In Chapter Three, Verbiest described a method to measure the Earth radius derived from Riccioli's Book Ten, Section Four, 75 which generically deals with several geographical Problems, Two to Ten exemplifying a number of alternatives to calculate the Earth's diameter. According to Riccioli, Clavius tried to improve Maurolycus' formulations, whom, in his Geometrica Practica, explained three different methods to calculate the Earth's diameter. Verbiest followed Riccioli's second method derived from one of Clavius's three methods.
Althought Verbiest's used as illustration a reprint from Riccioli's book, some passages of his accompanying text directly referred to Clavius's antecedant source.
Verbiest's dissertation on how to calculate heights, which precluded the knowledge of the Earth's radius, was equally derived from one of Riccioli's geographical problems entitled: Problema de distantia visus investiganda (Problem to Determine Visual Distance). 76
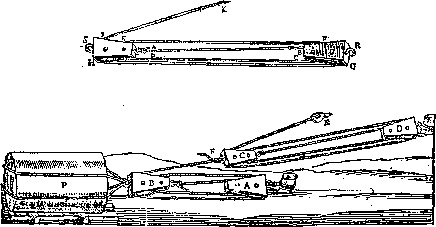
Trochlea-/ rum coniu-/ gatarum -, /Compostio, / quàtm ma-/ gnas vires/habeat (Multiple pulley).
In: CASATO, Paolo, Terra Machinis Mota Dissertationes / geometricœ, Mechanicœ / Physicœ, Hydrostaticœ [...]. Romæ, ex Typographia Ignatij de Lazaris.
After the geographical problems followed nautical problems which involved the charting of sailing between two places situated in locations different from one another, both in longitude and latitude.
This theoretical text is partially derived from Schall's Huntian yi shuo, particularly from the Qiu hai zhong zhou (Planning the Orientation for Ships at Sea). 77
Its ensuing, more practical section, dealing with a number of different problems to solve and a number of charts, was entirely transcribed from Adrianus Metius. 78
To this, Verbiest added another one indicating the ratio of the "great circle" (Equator) and the "small circles" (Meridians). This chart, probably originally printed in P. Apianus's Cosmographia, published in Koln, in 1574 (p. 16), later appeared - with a number of variants - in many Western works such as in those by Argoli, Riccioli, Metius, Hues, Stevin, and Schall's Hun tian yi shuo. 79 The direct source for both Schall's and Verbiest's charts is the one printed in Metius work, published in Amsterdam, in 1624. 80
[IV] In Chapter Four Verbiest points to the atmospheric conditions which must be taken into account to achieve accurate measurements. With regard to temperature and the degree of humidity, he described the apparatus of the thermometer and the hygrometer, two instruments new to China but already known in the Netherlands. Verbiest's study on the thermometer, the Yen qi tu shuo (Illustrated Dissertation on the Investigation of the Air), was separately published in 1671.
In the Ce tian zhu qi zhi fa (Method to Measure Atmospheric Conditions) Verbiest copied a number of refraction charts. The, water-air, water-glass, glass-air, glass-water and air-glass charts were taken from Vitello and the air-water chart from Maignan; all these charts also appearing under the title Tabulœ anaclasticœ seu refractionem (pp.642-662), in Riccioli's Almagestum novum. Vitello's charts appeared inserted in a section on Optics, in Kircher's Ars magna lucis et umbrœ, published in Rome, in 1646.
Trochlea multiplices (Multiple pulley).
Diagram by Paolo Casato, interpreted by Nicole Halsberghe.
Verbiest seems to have partially reproduced Kircher's charts implementing them in a more complete rendering but is also possible that he might have directly copied charts from Vitello's work Perspectiva (also named: Peri optikes).
Verbiest's adaptation of a chart from Maignan's Dioptrice horaria's Book Four, Proposition 45 (p.646), published in Rome, in 1648, is not present in Kircher's work, but Riccioli copied this chart in his De refractionibus, Book Ten, Section Six (p.464) comparing it to the table Maignan made with the help of relations directly based on experiments, and for which the Vitello ratio was also fundamental. This meant that Verbiest did not necessarily have to consult the original printed sources for making his charts, but that, ultimately, Riccioli's work might have been sufficient for computing his charts.
TAB VL Æ ANACLASTIC Æ
S E V
REFRACTIONVM.
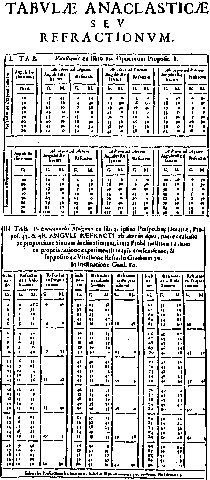
Refraction charts.
In: RICCIOLI, Giovanni Battista, Almagestum novum astronomiam veterem novamque complectens observationibus aliorum, et propriis novisque theorematibus, problematibus, ac tabulis promotam, in tres tomos distributam quorum argumentum sequens pagina explicabit, 3 vols., Bononiae, 1651, bk. 10, sect.6, pp.664-665.
In the Matter Optica Verbiest discourses on his theories about the spectrum, the rainbow and the halo.
For the spectrum Verbiest follows the text of Athanasius Kircher's De multiplici varietate colorum. 81 Kircher's classification of colours in zhen shi (veros) and huan wang (apparentes) establishes that he, most probably, copied Kircher's spectrum illustration (p.49), althought his introduction text on colours and its description of the rainbow are taken from Vagnoni's Kongji gezhi. 82
Verbiest proceeds with an exposition on the Chinese hou qi pseudo-science, possibly in deference to Yang Guangxian who not only defended this system but also attempted to give it a new impulse. Together with some references in coeval petitions to the Kangxi Emperor (KX 8:6:29), Fr. Gabriel de Magalhães' writings83 provided interesting material on contemporary circumstances peripheral to this topic. Both these sources and Verbiest's duplication of this text's passage on the hou qi, in his Bu de yi bian, prove his refutation of this system.
After dealing with optical matters he approaches some measuring methods. He starts by discussing a method for measuring the height of the clouds in the sky (pp.19a-21b), which is completely adapted from Riccioli's work, where this author vividly records a practical measurement performed together with Fr. Grimaldi. Verbiest's illustration (no110) corresponds to that of Riccioli. 84 He ended this section with the Ce shui fa (Method for Measuring Water) which was, once again, adapted from Riccioli's Almagestum novum. 85
In the last part of Chapter Four Verbiest tackled the pendulum, which he described as chui xian qiu (hanging-rod-ball instrument); a contraption reduced to its essential components. 86 Verbiest's pendulum, constituted by a heavy ball hung from a thin rope on a metal rod, closely corresponding to Riccioli's protoype and to the later type described by A. Heckscher87as "Das mathematische Pendel" or "funependulum" (string pendulum). Riccioli, having pioneered the systematic testing of pendular oscillations88 was rather favourable to Verbiest inserting this type of pendulum in his descriptive analysis. Both Verbiest's pendulum's components and its explanatory charts, as well as the accompanying problems on oscillation are exactly the same as in Riccioli's work. 89 For this reason, Verbiest's reference in this Chapter to a "Yuan xi zhi li xue ming ji" ("a famous calender expert from the distant West") was probably referenting to Riccioli, as this author mentioned that the idea of the pendulum had occured to him well before he had read Galilei's books.
With the excursus on the pendulum Verbiest concluded his Chapter Four.
[V-XIV] Chapters Five to Fourteen deal with assorted charts and a include a Catalogue of Stars. 90
Spectrum chart.
In: KIRCHER, Athanasius, Ars magna Lucis et Umbrœ [...], Romæ, 1646, p.49.
Spectrum chart.
In: KIRCHER, Athanasius, Ars magna Lucis et Umbrœ [...], Romæ, 1646, p.55.
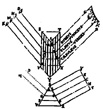
Spectrum chart.
In: AGUILONIUS, Franciscus, Opticorum libri sex[...], Antuerpiæ, 1613, p.55.
CHINESE GLOSSARY
bian quan si fen zhi yi 篇圈四份之一
Biao du shuo 表度説
Bi li gui jie 比例規解
Bu de yi bian 不得已辨
Ce hengxing chidao jing wei du zhi qi 測恆星赤道經緯度之器
Ce hengxing xiang ju zhi ji 測恆星相距之器
Ce liang quan yi 測量全義儀
Ce qi da bei 測器大備
Ce tian zhu qi zhi fa 測天諸氣之法
Ce shui fa 測水法
Ce yan ji lue 測驗紀略
Chidao jing wei jian yi 赤道經緯簡儀
Chidao jing wei quan yi 赤道經緯全儀
Chui xian qiu 垂線球
Fan zhu tong yi xian yong la zuo zhe yi zhi shi yang 凡鑄銅儀先用躐作者儀之式様
gao hu 高弧
Gewu qiongli 格物窮理
Guo Shoujing 郭守敬
hou qi 候氣
huan wang 幻妄
huang la 黄躐
Huntian yi shuo 渾天儀説
Hunyi 渾儀
jian yi 簡儀
Ji he yuan ben 幾何原本
jinye 金葉
Kong bu 工部
Kongji ge zhi 空際格致
Kui biao 窺表
Kuifa 窺法
Kunyu tu shuo 坤輿圖説
li chun 立春
Li Wei 李(霨)蔚
Li yun xuan 立連環
lun xiang lian 輪相連
Qin ding yi xiang kao cheng 欽定儀象考成
Qiu hai zhong zhou 求海中舟
Rizhan lizhi 日占曆指
songxiang 松香
Shangwu yin shu guan 商務印書館
Tian kong kai wu 天工開物
tian qiu 天球
tie 鐵
tong 銅
Tu Hai 圖海
wei hu 緯弧
Wen yuan ge (si ku quan shu) 文淵閣《四庫全書》
wu zhi ben zhong 物之本重
Xin fa biao yi 新法表異
Xin fa chidao jing wei yi 新法赤道經緯儀
Xin fa diping jing wei yi 新法地平經緯儀
Xin fa huangdao jing wei yi 新法黄道經緯儀
Xin fa li yin 新法曆引
Xin fa suan shu 新法算書
Xin zhi lingtai yixiang zhi 新制靈臺儀象志
Xin yi qing zhong bili fa 新儀輕重比例法
Xi shi Jialile zhi fa 西士嘉理勒之法
Yan qi tu shuo 驗氣圖説
Yi qi tu shuo 儀器圖説
Yixiang tu 儀象圖
Yixiang zhi 儀象志
yuan 元
Yuan xi qi qi tu shuo 遠西奇器圖説
Yuan xi zhi li xue ming jia 遠西之曆學名家
zhen shi 眞實
Zhong jie 重解
NOTES
1 GOLVERS, Noël, TheAstronomia Europaea of Ferdinand Verbiest, SJ. (Dillingen, 1687) in: "Monumenta Serica Monograph Series", Nettetal, Steyler Verlag, (28) 1993, p.15ff.
2 Ibidem., p.92
3 Ibidem., p. 250; JOSSON, H. - WILLAERT, L., Correspondance de Ferdinand Verbiest S. J., Directeur de l'Observatoire de Pékin, Bruxelles, Palais des Académies, 1938, p.181;
4 GOLVERS, Noöl, op. cit., p.251
5 HUCKER, Charles, A Dictionary of Official Titles in Imperial China, standford/California, 1985, no3462.
6 Chap. l, p. 22a.
7 BRAHE, Tycho, Ed. DREYER, J. L. E., Tychonis Brahe Dani Opera omnia, København, Hauniæ, In Libraria Gyldendaliana/Typis Nielsen & Lydiche (Axel Simmelkær), 1913-1929; BRAHE, Tycho, Astronomiae Instauratae Mechanica, Trans. RAEDER, H. -STRöMGREN, E. B., København, I Kommission hos ejnar Munksgaard, 1946, p. 53ff [lst edition: Impressum Wandesburgi, [...] Propria authoris typographia: Waandesburgi in arce Ranzoviana prope Hamburgum sita [...], 1598].
8 The armillary instrument used by Hipparchus and the Ptolemaic armillœ later reconstructed by Regiomontanus.
NOLTE, Fr., Die Armillarsphäre: Abhandlungen der Geschichte der Naturwissenschaften und der Medezin, Erlangen, M. Mencke, 1922, ill.2 -The armillary instrument used by Hipparchus and the Ptolemaic armillœ later reconstructed by Regiomontanus.
9 BRAHE, (1913-1929), op. cit., vol. 5, p.55 - "Consequently we did not use this kind of armillary instrument very often, and particularly not when the greatest precision was required. We have preferred to invent other equatorial armillae which are free from this error, an error which it is impossible to remove by the use of larger zodiacal armillae, since the weight of the armillae then becomes that much greater". BRAHE, (1946), op. cit., p.55 - For the description of his instruments and scientific work.
10 HEVELIUS, J., Machinae coelestis, pars prior, organographiam, sive instrumentorum astronomicorum omnium quibus auctor hactenus sidera rimatus, ac dimensus est, accuratam delineationem, et descriptionem, plurimis iconibus [...]", Gedani, 1673, p.89.
11 JOSSON, H. - WILLAERT, L., op. cit., pp. 181-182.
12 Comp. RHO, Johannes, Ce liang quan yi (Complete Treatise on Measurements), Rev. by SCHALL, Adam, with collaboration of Chinese astronomers, 1631, in: "Xin fa suan shu" ("Treatises on Mathematical Astronomy According to the New Methods"), chap. 96, p. 745, {SKQS789}.
Ce hengxing chidao jing wei du zhi qi (Instrument for Measuring the Equatorial Coordinates of the fixed Stars.), in: "Xin fa suan shu", chap. 56, {SKQS 788}.
14 BRAHE, (1913-1926), op. cit., vol. 5, p.60.
15 Xin fa chidao jing wei yi (New Method of the Equatorial Instrument), (1631), in: "Xin fa suan shu", chap. 96, p.743, {SKQS 789}.
16 BRAHE, (1913-1926), op. cit., vol. 5, p.64.
17 Ibidem., p.60
18 Ibidem., p.59
19 Chap. l, p. 9b: 東西游轉,橫相切於赤道之經圈地
20 BRAHE, (1913-1926), op. cit., vol. 5, p.57.
21 Chap. 1, p.2b- Diping jingwei yi (Altazimuthal instrument), divided in an jing yi 經儀 (azimuth instrument) and wei yi 緯儀(altitude instrument).
22 RHO, Johannes, op. cit., chap. 96, p.740, {SKQS 789}.
23 KÖGLER, Ignatius, Qin ding yi xiang kao cheng, (1742), pp. 6b-7a,26, {SKQS 793}.
24 NEEDHAM, Joseph, The hall of heavenly records, Korean astronomical instruments and clocks 1380-1780, Cambridge, Cambridge University Press, 1986, p.59.
25 Chap. l, 14b-15a
26 JOURDAIN, M., Mémoire sur les Instruments Employés à l'Observatoire de Pékin, in: "Magasin Encyclopédique ou Journal des Sciences", Paris, 1809, vol.4, pp. 43-101; SEDILLOT, L. A., Mémoires sur les Instruments Astronomiques des Arabes, in: "Mémoires Présentés par Divers Savants à 1'Académie Royale des Inscriptions et Belles-Lettres de l'Institut de France", Paris, Institut de France, ser. I, vol. 1, p.201 - The new instruments of the Maragha Observatory (ca 12161) are attributed to Nasir al-din or his friend Mu'ayyad al-din al-Urdi al Dimisqi. We followed the translation of the Arabic Mss. by M. Jourdain and L. A. Sédillot.
27 See: SCHALL, Adam, Xin fa li yin, (ca1635), in: "Xin fa suan shu", chap. 97, p. 749, {SKQS 789} - This was also announced in a memorial to the throne, dated the 13th September 1629, by Xu Guangxi.
See: HASHIMOTO, Keizo, Hsü Kuang-Ch'i and Astronomical reform - The process of the Chinese Acceptance of Western Astronomy, Kansai, Kansai University Press 1988, p.217.
Also see: SCHALL, Adam, Xin fa biao yi, (ca1645), in: "Xin fa suan shu", chap. 100, p.814, {SKQS 789} -For the same new instruments.
28 BRAHE, (1913 1926), op. cit., vol. 5, p.12.
29 Ibidem., p.32 - This bench-model was illustrated as in VERBIEST, Ferdinand, Liber Organicus, 1677-1678, i11.5.
30 Chap. l, 16b: 弧以內象限空餘之地爲扁龍以充其內
31 BRAHE, (1913-1926), op. cit., vol. 5, p.33.
32 JOSSON, H. - WILLAERT, L., op. cit., pp. 179-184.
33 See: Note 27 supra.
34 Ce heng xing xiang ju zhi ji, in "Xin fa suan shu", chap. 56, p. 974, SKQS 788. 測恆星象聚之極
35 BRAHE, (1913-1926), op. cit., vol. 5, p.73.
36 Ibidem., p.25 - "The astronomical sextant instrument, which is particularly convenient in use, was first invented by myself, about twenty years ago. I have given it this name, because its circumference comprises one-sixth of a circle."
37 HEVELIUS, J., op. cit., p. 84.
38 Chap. l, 22a: 古用三稜角形之法而右左上下之既堅固亦輕巧,則用以合天使之彼此不相反也,水左右皆有細雲,彼此相連蓋藉之以堅固全儀者也
39 Chap. 1, "Method of Use of the Sextant".
40 BRAHE, (1913-1926), op. cit., vol. 5, p. 68.
41 Ibidem., p.72.
42 VENTURI, P. Tacchini, Opera storiche del P. Matteo Ricci, S. J., (2 vols.), p.60
43 BÉRNARD-MAÎTRE, Henri, L'apport scientifique du père Matthieu Ricci à la Chine, Tientsin, Hautes Études, 1935, p. 38
44 GÜNTHER, Siegmund, Erd-und Himmelgloben, ihre Geschichte und Konstruktion, nach dem italienischen Matteo Fiorinis frei bearbeitet, Leipzig, G. B. Teubner, 1895, pp. 497-500.
45 HASHIMOTO, Keizo, op. cit., p. 217.
46 SCHALL, Adam, (ca1635), op. cit., chap. 97, p.749, {SKQS 789}.
47 SCHALL, Adam, (ca1635), op. cit., chap. 100, {SKQS 789}.
48 Cf. Notes 46,47 supra: Tian qiu天球
49 SCHALL, Adam, Hun tian yi, (1636), in: "Xin fa suan shu", chap.5, p.85, SKQS 788.
50 VÄTH, A., Johann Adam Schall von Bell S. J., Missionar in China, Kaiserlicher Astronom und Ratgeber am Hofe von Peking, 1592-1666", Köln, J. B. Bachem, 1933.
51 BARTOLI, Daniel, Dell'Istoria della Compagnia di Gèsu, Piacenza, Tip. del Majno, 1819-1821, part. I, vol.4. chap. 225, p.483
52 Huntian yi shuo, in: "Xin fa suan shu", chap. 16, {SKQS 788}.
53 Ibidem., n.4; GOLVERS, Noël, op. cit., pp. 250-251, n.21.
54 HUES, Robertus, Tractaet Ofte Handelinge van het gebruijck der Hemelscher ende Artscher Globe. [...] Door I. Hondiu, Amstelredamm, Michiel Colijn, 1612 [1st edition: Enghelant, Iodocvs Hondinvs, 1593], p.8
55 BRAHE, (1913-1926), op. cit., vol. 5., p.104.
56 REGIOMONTANIS, Ioannis M., Epytoma Joanis de möte regio In almage∫tu ptolomeї, Venetiis, 1496, liber 8, propositio 2.
57 RHO, Johannes, op. cit. p. 725.
58 HALSBERGHE, Nicole, Quotations from the works of A. Schall in the Yi xiang zhi of F. Verbiest, in: "INTERNATIONAL SYMPOSIUM ON THE OCCASION OF THE 400TH ANNIVERSARY OF THE BIRTH OF J. A. SCHALL VON BELL, S. J. (°1592-†1666)", May 1992, Sankt Augustin -- Oral communication.
59 Idem.
60 VERBIEST, Ferdinand, Xi chao dang an (Collection of Petitions and Memorials), BNP: Ms. Chinois, 1329, Xichao dang an, vol.1, pp. 33-34.
61 RHO, Johannes, op. cit., p.735--The same problem is also discussed by Johannes Rho and Adam Schall.
62 BRAHE, (1913-1926), op. cit., vol. 5, p.41.
63 This system will be discussed more detailed in my elaborate study of the Xin zhi lingtai yixiang zhi, in preparation.
64 GALILEI, Galileo, Discorsi e Dimonstrazioni Matematiche Intorno а due Nuove Scienze, Leida 1638, pp. 108-149; GAILILEI, Galileo; Trans. SALVIO, A. de, Dialogues concerning the two new sciences by Galileo Galilei, in: "Great Books of the Western World", Chicago, The University of Chicago, (28)1952, pp. 138.
65 RHO, Johannes; Rev. SCHALL, Adam, (Explanation of the Compasses of Proportions), (1600), in: "Xin fa suan shu", chap. 21, p.317, {SKQS 788}.
66 URSIS, Sabatino de, Biao du shuo (Explanation of the Gnomon), (1614), in: "Xin fa suan shu", p.815, {SKQS 810}.
67 MERSENNE, M., Les Mйchaniques de Galilйe", Paris, H. Gvenon, 1634, p. 26 -- Galilei's work was published for the first time by M. Mersenne: "To find out the causes and reasons of everything that happens to machines of which we have to explain the way of working, because every science has definitions and principles [...]. Well then as machines generally serve to transport heavy things we start with the definition of weight which is also called gravity"
68 PRADO, Hieronymous-VILLALPANDO, Joannis-Batistæ, S. J., In Ezechielem explanationes et apparatus urbis et templi Hierosolymitani commentariis et imaginibus illustratus, 3 vols., Romae, Ciacconii, 1596-1604, vol. 3, chap. 6, (door Villalpando) -- For true basics of A. Kircher's exposition.
69 BETTINI, M., Apiaria universae philosophiae mathematicae in quibus paradoxa et nova pleraque machinamenta ad usus eximios traducta [...], Bononiae, Jo. Bapt. Ferronii, 1642, vol.1, apiaria 4, proggym. 2, p.34.
70 CASATO, Paolo, Terra machinis mota eiusque gravitas et dimensio dissertationes ducœ, Romæ, Typis Haeredum Corbelletti, 1655, pp. 5-6.
71 CAUS, Salomon de, Les Raisons des forces mouuantes avec diuerses Machines tant vtilles que plaisantes. Aus quelles sont adioints plusieurs desseings de grotes et fontaines[...], Fancfort, Jan Norton, 1615, p.8.
72 RICCIOLI, Giovanni Battista, Almagestum Novum Astronomiam Veterem Novamque Complectens Observationibus Aliorum, et Propriis Nouisque Theorematibus, Problematibus, ac Tabulis Promotam, in Tres Tomos Distributam Quorum Argumentum Sequens Pagina Explicabit, Bononiæ, ex. Typographia Hæredis Victorii Benatii, 1615, part. II, vol. 1, pp. 328-330.
73 KIRCHER, Athanasius, Magnes Siue de Arte Magnetica opus tripartitvm, Quo Prœterqvam Qvod Vniversa Magnetis Nat√ra, Eivsqve In Omnibvs Artibvs & Scientijs v∫us noua Methodo explicetur, [...], Coloniæ Agrippinæ, apud Iodocvm Kalcoven, 1643 [editio secunda], pp. 347-37.
74 RHO, Johannes, Rizhan lizhi, Rev. SCHALL, Adam, Ding nan bei xian (Fixing the North-South Line), in: "Xin fa suan shu", chap. 24, pp. 367-368 -- The three methods. See: KЦGLER, Ignatius, Yu zhi li xiang kao cheng, in: "Xin fa suan shu", p.101-104. -- Where these methods are equally explained.
75 METIUS, Adrianus, De Genuino Usu Utriusque Globi Tractatus. Adjecta Est nova Sciatericorum, & Arts Navigandi Ratio Novis Instrumentis, & Inventionibus Illustrata, Amstelodami, Guiljelmum Janssonium Caesium, 1624, sium,1624,part ll,bk,.10,sec,4, p.587.
76 Ibidem., p.1, p.65.
77 Qiu hai zhong zhou, in: "Xin fa suan shu", chap. 4, pp. 295-298, SKQS 788.
78 METIUS, op. cit., pp. 65-80.
79 SCHALL, Adam, (ca1636), op. cit., chap. 4, 298, {SKQS 788}.
80 METIUS, op. cit., pp. 65-80.
81 KIRCHER, Athanasius, Ars magna Lucis et Umbraœ in decem Libros digesta [...], 10 vols., Romae, Sumptibus Hermanni Scheus, ex. Typographia Ludouici Grignani, 1646, pp. 48-50.
82 VAGNONI, A., Kongji Gezhi Xia, 1633, pp. 16-17 --Namely his Kong yi si (Study on Colours) and his Hongni (Study on the Rainbow).
83 PIH, Irene, Le pиre Gabriel de Magalhгes; un jesuite Portugais en Chine au XVIIe siиcle, Paris, Fundaзгo Calouste Gulbenkian, 1979, pp. 193-195.
84 RICCIOLI, Giovanni Battista, op. cit., part I, bk. 2, p. 82.
85 Ibidem., part I, bk. 10, p.594. -- Riccioli treaded it as "Montium altitudinem metiri more antiquiorum geometrarum, duplicem tamen fallaciam continente" ("Measuring the height of mountains on the way of the old surveyors, that also included a double fault").
86 He noticed that the Jesuits if they don't have an adequate Chinese translation for technical terms always reduce the translation to the essential components.
87 HECKSCHER, A., Historische Herleitung der Pendelgesetze, Leipzig, Archiv fьr Geschichte der Naturwissenschaften und der Technik, 1914, vol. 5, i11.3.
88 BERTHOUD, F., Histoire de la Mesure du Temps par les Horloges, Paris, Imprimerie de la Rйpublique, 1802, vol.1, p.85.
89 RICCIOLI, Giovanni Battista, op. cit., part II, bk. 20, pp. 84-91
90 The complete translation of, annotations and comments on Verbiest's Xin zhi lingtai yixiang zhi was the subject of my Ph. D dissertation.
*Ph. D in Oriental Studies and Sinology from the Catholic University of Leuven: Thesis dissertation: The complete translation of Verbiest's Xin Zhi Lingtai Yixiang Zhi, with annotations and commentaries. Professor of Art in the High School of Art and Architecture, Gent, and lecturer in the Department of Sinology, University of Leuven.
start p. 213
end p.